25 Two cars that may collide at 12:30 PM
Problem 25
One city E, is 20 miles north and 20 miles east of another city, F. At noon a car starts south from E at 40 mi/hr, at 12:10 PM, another car starts east from F at 60 mi/hr. Find the rate at which the cars approach each other between 12:10 PM and 12:30 PM. What happens at 12:30 PM?
- Read more about 25 Two cars that may collide at 12:30 PM
- Log in or register to post comments

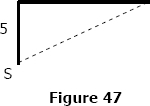 A boy on a bike rides north 5 mi, then turns east (Fig. 47). If he rides 10 mi/hr, at what rate does his distance to the starting point S changing 2 hour after he left that point?
A boy on a bike rides north 5 mi, then turns east (Fig. 47). If he rides 10 mi/hr, at what rate does his distance to the starting point S changing 2 hour after he left that point?