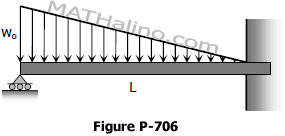
Problem 706 | Solution of Propped Beam with Decreasing Load
Example 03
The propped beam shown in Fig. P -706 is loaded by decreasing triangular load varying from wo from the simple end to zero at the fixed end. Find the support reactions and sketch the shear and moment diagrams