fully restrained beam

Summary for the value of end moments and deflection of perfectly restrained beam carrying various loadings. Note that for values of EIy, y is positive downward.
 

Case 1: Concentrated load anywhere on the span of fully restrained beam

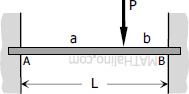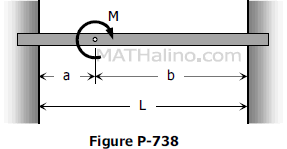
End moments
$M_A = -\dfrac{Pab^2}{L^2}$

$M_B = -\dfrac{Pa^2b}{L^2}$
 

Value of EIy
$\text{Midspan } EI\,y = \dfrac{Pb^2}{48}(3L - 4b)$

Note: only for b > a

Problem 737
In the perfectly restrained beam shown in Fig. P-737, support B has settled a distance Δ below support A. Show that M_B = -M_A = 6EIΔ/L².
 

Problem 736
Determine the end shears and end moments for the restrained beam shown in Fig. P-736 and sketch the shear and moment diagrams.
 

Problem 734
Determine the end moments for the restrained beams shown in Fig. P-734.
 

Problem 703
Determine the end moment and maximum deflection for a perfectly restrained beam loaded as shown in Fig. P-730.