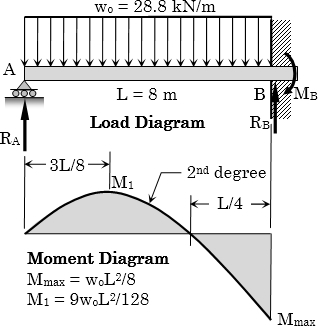
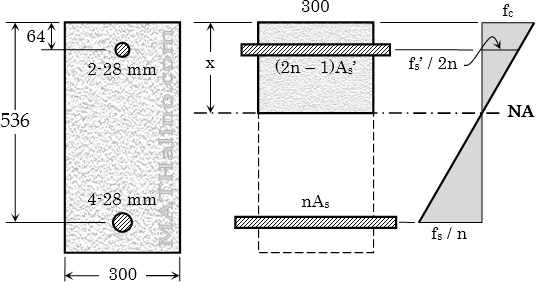
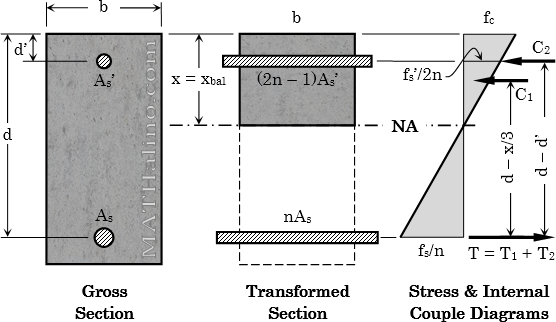
Example 03: Finding the Number of 32-mm Steel Bars for Doubly-Reinforced Concrete Propped Beam
Problem
A propped beam 8 m long is to support a total load of 28.8 kN/m. It is desired to find the steel reinforcements at the most critical section in bending. The cross section of the concrete beam is 400 mm by 600 mm with an effective cover of 60 mm for the reinforcements. f’c = 21 MPa, fs = 140 MPa, n = 9. Determine the required number of 32 mm ø tension bars and the required number of 32 mm ø compression bars.