March 2017

Problem
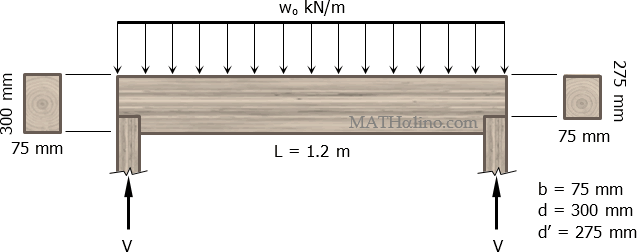
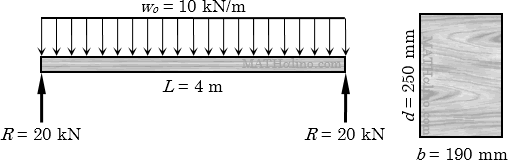
A 75 mm × 150 mm beam carries a uniform load w_o over the entire span of 1.2 m. Square notches 25 mm deep are provided at the bottom of the beam at the supports. Calculate the safe value of w_o based on shear alone.

Problem
A beam is built up by nailing together 25 mm thick planks to form a 200 mm × 250 mm box section as shown. The nails are spaced 125 mm apart and each can carry a shearing force of up to 1.3 kN. The beam is simply supported for a span of 3.6 m and to carry a concentrated load P at the third point of the span. The allowable shearing stress of the section is 0.827 MPa.
 

1. Determine the largest value of P that will not exceed the allowable shearing stress of the beam or the allowable shearing force of the nails.
2. What is the maximum flexural stress of the beam for the load P computed in Part (1)?

Problem
A concentrated load P is carried at midspan by a simply supported 4-m span beam. The beam is made of 40-mm by 150-mm timber screwed together, as shown. The maximum flexural stress developed is 8.3 MPa and each screw can resist 890 N of shear force.
 

1.   Determine the spacing of screws at A.
2.   Determine the spacing of screws at B.

Problem
A beam 100 mm wide is to be loaded with 3 kN concentrated loads spaced uniformly at 0.40 m on centers throughout the 5 m span. The following data are given:

1. Find the depth d considering bending stress only.
2. Determine the depth d considering shear stress only.
3. Calculate the depth d considering deflection only.

Problem
Steel and aluminum plates are used to reinforced an 80 mm by 150 mm timber beam. The three materials are fastened firmly as shown so that there will be no relative movement between them.
 

Given the following material properties:

Find the safe resisting moment of the beam in kN·m.
 

Problem
A wooden log is to be used as a footbridge to span 3-m gap. The log is required to support a concentrated load of 30 kN at midspan. If the allowable stress in shear is 0.7 MPa, what is the diameter of the log that would be needed. Assume the log is very nearly circular and the bending stresses are adequately met. Neglect the weight of the log.
 

Problem
A timber beam 4 m long is simply supported at both ends. It carries a uniform load of 10 kN/m including its own weight. The wooden section has a width of 200 mm and a depth of 260 mm and is made up of 80% grade Apitong. Use dressed dimension by reducing its dimensions by 10 mm.

1. What is the maximum flexural stress of the beam?
2. What is the maximum shearing stress of the beam?
3. What is the maximum deflection of the beam?