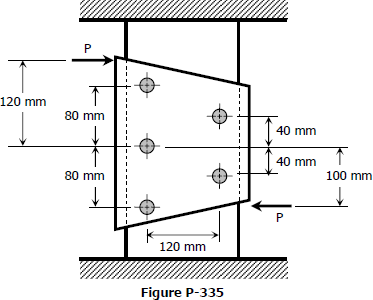
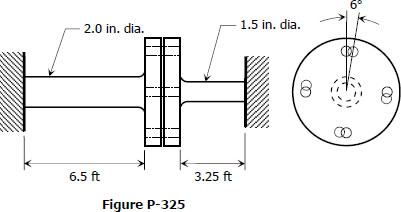
Solution to Problem 344 | Helical Springs
Problem 344
Determine the maximum shearing stress and elongation in a bronze helical spring composed of 20 turns of 1.0-in.-diameter wire on a mean radius of 4 in. when the spring is supporting a load of 500 lb. Use Eq. (3-10) and G = 6 × 106 psi.
- Read more about Solution to Problem 344 | Helical Springs
- Log in or register to post comments