Solution to Problem 617 | Double Integration Method Jhun Vert Thu, 04/23/2020 - 04:24 pm

Problem 617
Replace the load P in Prob. 616 by a clockwise couple M applied at the right end and determine the slope and deflection at the right end.
 

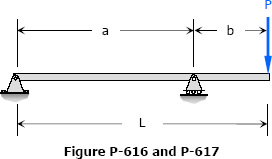
Method of Superposition | Beam Deflection Jhun Vert Thu, 04/23/2020 - 03:17 pm

The slope or deflection at any point on the beam is equal to the resultant of the slopes or deflections at that point caused by each of the load acting separately.
 

Rotation and Deflection for Common Loadings

Case 1: Concentrated load at the free end of cantilever beam
superposition-case-01.jpgMaximum Moment
$M = -PL$

Slope at end
$\theta = \dfrac{PL^2}{2EI}$

Maximum deflection
$\delta = \dfrac{PL^3}{3EI}$

Deflection Equation ($y$ is positive downward)
$EI \, y = \dfrac{Px^2}{6}(3L - x)$
 

Area-Moment Method | Beam Deflections Jhun Vert Thu, 04/23/2020 - 03:03 pm

Video

Another method of determining the slopes and deflections in beams is the area-moment method, which involves the area of the moment diagram.
 

Deviation and Slope of Beam by Area-Moment Method