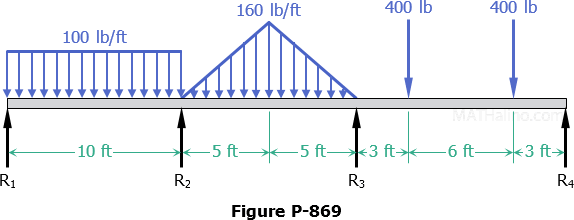
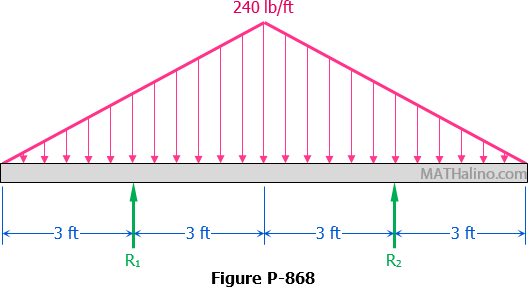
Kinematics - Two particles release from same height ...
Good afternoon (from Europe) !
The given solution of mentioned problem is wrong.
There must be some initial velocity of particle "A" moving downwards along slope.
Particle "\"B\"": t_B=√(2h/g)
Particle "\"A\" ": L=v_(A,i) t+a t^2/2=v_(A,i) √(2h/g)+gsinα (√(2h/g))^2/2=v_(A,i) √(2h/g)+h^2/L
v_(A,i)=L(1-h^2/L^2 ) √(g/2h)=20*(1-〖12〗^2/〖20〗^2 ) √(9.81/(2*12))=8.18 m/s
The result meets our expectation.
Best regards !
congestus