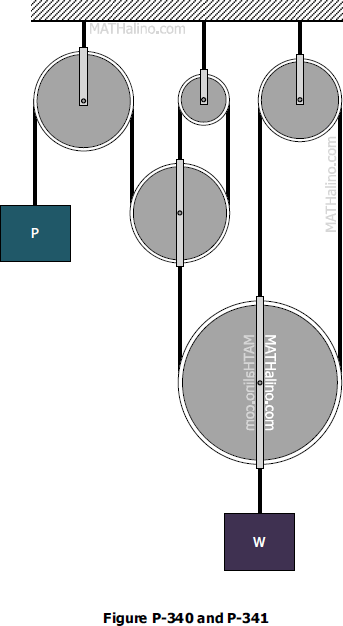
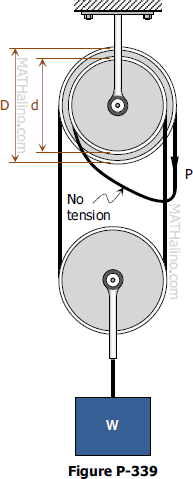
Problem 07 | Separation of Variables
Problem 07
$y' = x \exp (y - x^2)$, when $x = 0$, $y = 0$.
Solution 07
Click here to expand or collapse this section
$\dfrac{dy}{dx} = x e^{y - x^2}$
- Read more about Problem 07 | Separation of Variables
- Log in or register to post comments