Torsion
Torsion
Consider a bar to be rigidly attached at one end and twisted at the other end by a torque or twisting moment T equivalent to F × d, which is applied perpendicular to the axis of the bar, as shown in the figure. Such a bar is said to be in torsion.
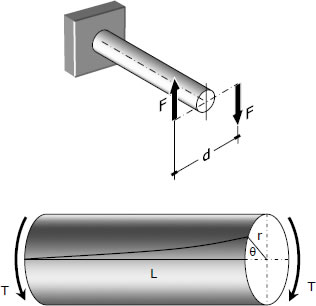
Back to top
Torsional Shearing Stress, τ
For a solid or hollow circular shaft subject to a twisting moment T, the torsional shearing stress τ at a distance ρ from the center of the shaft is
where J is the polar moment of inertia of the section and r is the outer radius.
For solid cylindrical shaft:
 J=π32D4
J=π32D4
τmax=16TπD3
For hollow cylindrical shaft:
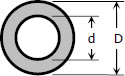 J=π32(D4−d4)
J=π32(D4−d4)
τmax=16TDπ(D4−d4)
Angle of Twist
The angle θ through which the bar length L will twist is
where T is the torque in N·mm, L is the length of shaft in mm, G is shear modulus in MPa, J is the polar moment of inertia in mm4, D and d are diameter in mm, and r is the radius in mm.
Power Transmitted by the Shaft
A shaft rotating with a constant angular velocity ω (in radians per second) is being acted by a twisting moment T. The power transmitted by the shaft is
where T is the torque in N·m, f is the number of revolutions per second, and P is the power in watts.
