Calculator Technique for Solving Volume Flow Rate Problems in Calculus

The following models of CASIO calculator may work with this method: fx-570ES, fx-570ES Plus, fx-115ES, fx-115ES Plus, fx-991ES, and fx-991ES Plus.

The following calculator keys will be used for the solution

Name	Key	Operation
Shift		`SHIFT`
Mode		`MODE`

Name	Key	Operation
Stat		`SHIFT → 1[STAT]`
AC		`AC`

This is one of the series of post in calculator techniques in solving problems. You may also be interested in my previous posts: Calculator technique for progression problems and Calculator technique for clock problems; both in Algebra.

Flow Rate Problem
Water is poured into a conical tank at the rate of 2.15 cubic meters per minute. The tank is 8 meters in diameter across the top and 10 meters high. How fast the water level rising when the water stands 3.5 meters deep.

Traditional Solution
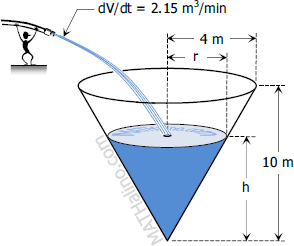$\dfrac{r}{h} = \dfrac{4}{10}$

$r = \frac{2}{5}h$

Volume of water inside the tank
$V = \frac{1}{3}\pi r^2 h$

$V = \frac{1}{3}\pi (\frac{2}{5}h)^2 h$

$V = \frac{4}{75}\pi h^3$

Differentiate both sides with respect to time
$\dfrac{dV}{dt} = \frac{4}{25}\pi h^2 \dfrac{dh}{dt}$

$2.15 = \frac{4}{25}\pi h^2 \dfrac{dh}{dt}$

When h = 3.5 m
$2.15 = \frac{4}{25}\pi (3.5^2) \dfrac{dh}{dt}$

$\dfrac{dh}{dt} = 0.3492 \, \text{m/min}$ answer

Solution by Calculator

Click here to show or hide the concept behind this technique

Understand why this calculator technique works
In Hydraulics, discharge or volume flow rate is given by the formula

$Q = vA$

Where
$Q$ = the discharge or volume flow rate
$v$ = velocity of flow
$A$ = cross-sectional area of flow

The equivalent of the above elements in Calculus are:
$Q = \dfrac{dV}{dt}$ where V is the volume and dV/dt is the volume flow (time) rates and

$v = \dfrac{dh}{dt} = \dfrac{dx}{dt} = \dfrac{dy}{dt} = \dfrac{ds}{dt}$ where h, x, y, s are distances and v is velocity.

Thus, the formula Q = vA can be written as

$\dfrac{dV}{dt} = \dfrac{dh}{dt}A$

which is the formula we are going to use in our calculator.

For the area A
The general prismatoid is a solid in which the area of any section, say A_y, parallel to and at a distance y from a fixed plane can be expressed as a polynomial in y not higher than third degree, or

$A_y = a + by + cy^2 + dy^3$

Common solids like cone, prism, cylinder, frustums, pyramid, and sphere are actually prismatoids in which any area parallel to a base is at most a quadratic function in height y. Thus,

$A_y = a + by + cy^2$

We can therefore use the Quadratic Regression in STAT mode of the calculator to find the area in relation with its distance from a predefined plane.

MODE → 3:STAT → 3:_+cX<sup>2</sup>

X	Y
0	0
10	π4²
5	π2²

AC → 2.15 ÷ 3.5y-caret = 0.3492 answer

To input the 3.5y-caret above, do
3.5 → SHIFT → 1[STAT] → 7:Reg → 6:y-caret

What we just did was actually v = Q / A which is the equivalent of $\dfrac{dh}{dt} = \dfrac{dV/dt}{A}$ for this problem.

Problem
Water is being poured into a hemispherical bowl of radius 6 inches at the rate of x cubic inches per second. Find x if the water level is rising at 0.1273 inch per second when it is 2 inches deep?

Traditional Solution
Volume of water inside the bowl
$V = \frac{1}{3}\pi h^2(3r − h)$

$V = \frac{1}{3}\pi h^2 [ \, 3(6) - h \, ]$

$V = \frac{1}{3}\pi (18h^2 - h^3)$

Differentiate both sides with respect to time
$\dfrac{dV}{dt} = \frac{1}{3}\pi (36h - 3h^2) \dfrac{dh}{dt}$

When h = 2 inches, dh/dt = 0.1273 inch/sec
$\dfrac{dV}{dt} = \frac{1}{3}\pi [ \, 36(2) - 3(2^2) \, ] (0.1273)$

$x = 7.9985 \, \text{in}^3\text{/sec}$ answer

Calculator Technique
MODE → 3:STAT → 3:_+cX<sup>2</sup>

X	Y
0	0
6	π6²
12	0

AC → 0.1273 × 2y-caret = 7.9985 answer

I hope you enjoy this post. Next time you solve problems involving flow rate, try to use this calculator technique to save time.

Calculator Technique for Solving Volume Flow Rate Problems in Calculus

More Blog Posts

Recent comments